research > theory > The Theoretical Eye
Anthony Auerbach

‘The Theoretical Eye’, paper by Anthony Auerbach published in the Journal of Art Historiography, no. 5, December 2011 together with the translation of Hubert Damisch’s ‘L’oeil théoricien’. An earlier version of this paper was presented at the symposium Hubert Damisch: Dialogues with Others, University of Amsterdam, 29 May 2009, organised by Sophie Berrebi and Eric De Bruyn.


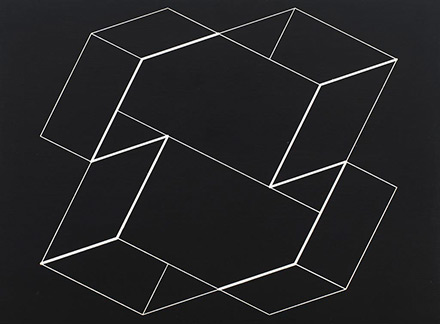
Figure 9: Josef Albers, Structural Constellation, c. 1950–60. Machine engraving on black vinylite mounted on board, 20.3 x 27.9 cm. Bethany, CT: The Josef and Anni Albers Foundation. Copyright: The Josef and Anni Albers Foundation (1976.8.1865/SC: 5).

The theoretical eye conjures images: the shiny pupil peering from the hole in Brunelleschi’s miniature of the Baptistry in Florence—that founding manifesto that designated and masked the location of the looker, and whose legend announces the origin of perspective; Dürer’s rebus-eyes inhabiting the apex of a visual pyramid and the spot which came to be known as the vanishing point; his metal alter-ego, a prosthetic eye, hammered into a wall, displacing the human subject that would trace the contour of appearance, or animating the automaton he made of Italian art theory; the globe suspended like an inverted balloon above Descartes’ philosophical avatar as if he were Tantalus; the apparatus of the pine kernel of the soul, rigged like a puppeteer; the not-image of the eye, as alluring as it is impossible, that Wittgenstein cited in asserting that ‘The subject does not belong to the world: rather it is a limit of the world’ [note 1]; the watery capsule of Freud’s anatomy of the soul in which the components of the ‘psychical personality’ seem to swim; the iridescent animal eye which appears on the school bench for dissection in honour of Descartes’ experiments. (Figs. 1–8, in-line)

‘The Theoretical Eye’, in the more personal French formula, ‘L’œil théoricien’, is also the headline Hubert Damisch chose for a short essay on an artist whose work appears obstinately abstract. [note 2] The title by itself (the eye, a theorist) is perhaps the shortest possible abbreviation of Damisch’s intellectual project. The topic, the work of Josef Albers, epitomises perhaps better than that of any other artist the reflexive qualities of artworks which Damisch has consistently emphasised: the artwork as the work on language. The text sets out against Freud, with a motto from Marx, to suggest how an œuvre which seems so determined to repudiate the notion of meaning that would have been recognisable to Freud—and to art history in so far as it shares Freud’s notion of interpretation—how such a body of work may nonetheless yield to analysis: an analysis that, like the clinical version, will thrive on ambiguity; a procedure that will declare its allegiance to Lacan by displaying its debts, on the one hand to (Freudian) psychoanalysis, and on the other hand to mathematics, in particular to geometry.

‘L’œil théoricien’, being abbreviated, is also overdetermined, and confronts the reader with a tantalising locution of the enigma posed by Albers’ work. Whereas a retrospective reading of the essay could amount (in Damisch’s words) to ‘interfering with his own history, his own past, his own obsessions, his own neurosis,’ I propose instead, and by way of a reply, to renew the encounter with Albers.

I propose an interpretation of a fraction of Albers’ work: possibly a monadic abbreviation, possibly a model, or just an example, but nonetheless, a distinct part. Structural Constellations designates a set of graphic works Albers began around 1950, [note 3] about the same time as he initiated the sequence of paintings Homage to the Square, for which he is best known. Both series occupied Albers until the end of his life. Pursuing each independently, Albers marked a decisive split between colour (in Homage to the Square, supported by only the faintest vestige of drawing) and graphic work (in Structural Constellations, anticipating only incision, construction or repetition), but he did not intervene in the perennial controversy over the primacy of line versus the primacy of colour. In granting each its autonomy, Albers did not attempt to adjudicate the matter nor aim to reconcile the advocates of drawing and painting.

Damisch’s hypothetical ‘geometry of colour’ acknowledges Albers’ indifference by interfering with the traditional terms of the dichotomy. The crossing of one term with the attribute of the other suggests an analogy between the operation of drawing and that of painting which perhaps holds only in Albers’s terms, [note 4] for the discrepancy Albers aimed to achieve and the ratio by which he measured his art are not the properties of lines or colours. [note 5] To stick with Albers’ graphic constructions is thus not necessarily to neglect the matter of his colour constellations, as the paintings might also be called. My procedure might not elucidate the ‘geometry of colour’, but it will say something about the predilection for such a formulation.

Albers’ reputation in the USA, where he found refuge after the Bauhaus was closed in 1933, was based mainly on his eminence as teacher and on his association with the defunct European avant gardes of the inter-war years, whose relicts were preserved in the Museum of Modern Art, one might say, as tribute to the American triumph. ‘American, born Germany’ reads the label today—Albers’ shortest biography. The late work of a foreigner and old-timer like Albers seemed neither historic nor topical to Albers’ contemporaries. The frustration, disappointment and bewilderment expressed by influential American critics certainly betray historical determinants, in so far as the discussion of abstract art was coloured by post-war and cold-war antagonisms, [note 6] but it is the structural determinants I would like to explore here: how Albers’ Structural Constellations function as a lure, drawing the customary discourse of art history and criticism into the entanglements of its own inheritance.

That function or quality of Albers’ art could be inferred, using routine art-historical technique, from the confused reception of Albers’ work. [note 7] But while a survey of the reception might indicate the knotty areas in the discourse, it doesn’t necessarily help bring Structural Constellations into view, still less bring off an interpretation. Moreover, if we really are dealing with a lure, we can expect the pitfalls it has prepared for us to remain hidden until it’s too late. If this drawing is a lure (Fig. 9, top of page), what artwork is not? The term ‘lure’, for now, at least, brackets the notion of ‘object’—in so far as a lure is not necessarily what it seems, and signals our own part in falling for it—whether it turns out to be an objet d’art, object of representation, or object of knowledge.

When Damisch asserts that an œuvre such as Albers’, which, he says, appears to ‘have broken with representation only to amuse itself with illusionistic games,’—when he asserts that such work ‘would not get any really satisfactory response within the language of art criticism or art history,’ he acknowledges something not quite satisfying about art history and criticism, fully aware that appealing beyond that language—specifically to psychoanalysis and to geometry—binds him to the language of art history and criticism, its traditions and its controversies. No discourse, except perhaps that of psychoanalysis, has lent more prestige to the discipline of art theory and interpretation, or fired more erudite disputes, than the discourse of geometry.

The Lacanian conjunction of geometry and psychoanalysis is itself mediated by art-historical and art-critical manoeuvres—indeed by artistic practices—and in truth owes more to them than to mathematics. Accordingly, Lacan is apt to cite the original, that is, the authority of a geometry book, rather than, say, the Unhappy Readymade Marcel Duchamp left out on a balcony for the wind to ‘choose its own problems, turn and tear out the pages,’ [note 8] he would rather display a topological diagram than ‘the one which disfigures measurement’ from the book Francis Picabia dedicated to ‘tous les docteurs neurologes en general’ [note 9]—just as art history and criticism would sooner cite first philosophy than the extravagant ingenuity of an Apollinaire [note 10] or the grandiloquent jargon of the -isms of art.

Damisch characterises Albers’ method as cunning, although, as he also remarks, the ingenuous simplicity of Albers’ means appears to conceal nothing. On what basis, other than experience, then, should one suspect, or could one predict mischief from this apparently minimal, almost artless art?—an art unannounced by any manifesto, asserting nothing a priori, advertising neither mysteries nor revelations. Adamant, rectilinear and symmetrical, Structural Constellations seem to have renounced every attribute of drawing except those associated with geometry. And what could be more beguiling than the appearance of geometry?

The duplicity of geometry’s appearance has a history which can be traced, as it were, to the origin of geometry. Euclid’s first postulate (to draw a straight line from any point to any point) is marked by the entanglement with graphic art that theory veiled in the elaboration of an autonomous, deductive science. Dissimulated would be the right word: sufficiently for geometry’s dependency on practical operations to be mainly overwhelmed by Euclid’s reputation as the author of the epitome of deductive reasoning, and for the originally visual notion of demonstration to emerge as the force of reason. Euclid’s Elements modelled the geometrical habit that philosophers adopted to lend a pattern of necessity to their speculations. Moreover, geometry’s dis-simulation, privileging the theoretical a priori over the representation of experience, underwrote the notion of a world of ideas, of essences uncoupled from appearances, assumed to be both prior and real, but accessible only to abstract thought.

Conversely (classically), the demonstrable proof of geometric theorems and their convincing application to music and astronomy were taken as signs of divine grace and true knowledge. The revolution Galileo got away with amounted to a cunning reversal of idealism. Substituting approximate mathematical descriptions for absolute explanations, Galileo seized on the compelling correlation of geometry and experience, but was careful to leave geometry on its divine pedestal, from which he would fashion the foundation of certainty for the new science. Philosophy, Galileo famously proclaimed, was to be read in the book of nature, ‘which stands continually open to our gaze,’ or rather, it was to be deciphered, since, he asserted, ‘It is written in the language of mathematics, and its characters are triangles, circles, and other geometric figures.’ [note 11] The appearance of such figures was thus the sign and seal of a divine contract and hence the true philosophy.

While the success of the new methods spelt the nemesis of the old authorities in physics, it seemed to affirm Euclid’s geometry as the true science of space. It did no harm to Euclid’s reputation if Newton hypostatised space as both logically prior and really existing, and thus absorbed geometry into physics, as merely that part of ‘universal mechanics which accurately proposes and demonstrates the art of measuring’. [note 12] Newton’s manoeuvre was convincing enough, it seems, to persuade the guileless Kant to bet his morals on geometry. Geometry, Kant thought, demonstrated what he called synthetic a priori judgements. His explanation renewed the contract with drawing: whereas a philosopher could proceed only analytically, by contrast, Kant says, the geometer, ‘at once begins by constructing a triangle [... he prolongs this side, divides this angle and so on]. In this fashion,’ Kant continues, ‘through a chain of inferences guided throughout by intuition, he arrives at a fully evident and universally valid solution of the problem.’ [note 13]

The immanent development of geometry as an abstract discipline scuppered Kant’s metaphysical arguments, not because an analogy between geometric theory and graphic construction does not hold, but because it could not be shown to be necessary—aside from the fact, as Freud remarked of his own work, that analogies decide nothing. [note 14] Nonetheless, Kant’s image of geometry endured. It remains an eminent witness to how, from the didactic function of drawing for Euclidean plane geometry, a class of graphic gestures emerged which were accepted as equivalent to abstract thought.

In the century after Kant, the scholarly discipline of geometry underwent a period of intense critical refinement which resulted in Euclidean geometry losing its status as the unique paradigm of epistemic certainty. Several other geometries were elaborated, each without contradiction, but by definition non-intuitive in Kantian terms, and making no claims on reality. While the recognition of non-Euclidean geometries led to a profound reassessment of the foundations of geometry as well as of the epistemic models of science, [note 15] at the same time, practical geometry underwent an enormous expansion. Drawing, and in answer to the growing needs of industrialisation, technical drawing in particular, earned a place alongside literacy and numeracy in the then emerging state apparatus of education, the national curriculum. The institutional and pedagogic justifications of the practice—its discipline in the Foucauldian sense—was however partly inherited from previous centuries. Nineteenth-century textbooks of geometrical or mechanical drawing, as it was variously called, combined epistemological wish-images such as Kant’s with notions indebted, on the one hand, to the academic tradition stemming from Alberti, and on the other hand, to the polytechnic tradition founded by Monge, resulting in an amalgam which was far from coherent.

The cornerstone of the École Polytechnique, Gaspard Monge’s Géométrie descriptive, is something of a ceremonial document. While the conceptual rigour and mathematical elegance that distinguished Monge’s teaching is credited with prompting the formal research to which we owe the modern mathematical discipline of projective geometry, it was honoured mostly only in name by Monge’s avatars in the classroom. Monge’s technique was based on Descartes’ method and accordingly made an impressive point of ‘the most intimate relations’ between analysis (algebra) and geometry: ‘every analytic operation,’ Monge says, can be regarded as ‘the script for a play in geometry’ (l’écriture d'un spectacle), of which drawing is the trace. [note 16] Descriptive geometry, one might say, is the spectacular form of rational objects. Or, this at least was the gist of descriptive geometry that was transmitted in the nineteenth-century teaching of technical drawing—not entirely without justification, but usually without conceptual or mathematical elaboration.

It should not be forgotten that the modernists of the first decades of the twentieth century all had a nineteenth-century education. Nor is it an accident that the first insults hurled at the artistic practice which came to be known as cubism were expressed in geometric terms. The rhetoric of geometry, moreover, provided the defenders and promoters of cubism with a ready-made vocabulary in which they could respond, and thus align their struggle with well-rehearsed disputes in French art theory. By the time an increasingly freewheeling debate about the merits of cubism, and by extension the fate of ‘l’ésprit géométrique’, reached a peak of excitement and confusion fuelled by mathematical rumours and a splintering rivalry for mantle of the avant garde, cubist paintings must have looked decidedly shabby and trivial in contrast with the universal mission advertised by the talk about geometry.

The polemics against perspective launched by the modernists (to which Erwin Panofsky responded in the 1920s) depended as much on the didactic justifications of technical drawing as on Alberti’s original cunning in bringing painting under the aegis of geometry.

Although Alberti had opened his treatise On Painting with a literary homage to Euclid, and echoed the legendary motto of the Platonic academy in stating, ‘It would please me if the painter were as learned as possible in all the liberal arts, but first of all I desire that he know geometry,’ [note 17] it was not actually by teaching geometry that Alberti sought to achieve his aim of establishing the prototype of the modern freelance artist as an educated man, worthy of the respect of high society, and the purveyor of portable cultural goods, specifically rectangular pictures.


Figure 10: A drawing apparatus from Albrecht Dürer, Underweysung der Messung, second edition, 1538. Nuremberg. Dürer demonstrates the device Alberti called a ‘veil’. The screen and corresponding grid on the artist’s drawing paper are repeated for the viewer in the pattern of windows looking over a landscape.

Alberti suggests how the geometry of appearance can be reduced to triangles the eye would measure ‘as with a pair of compasses’ (46), and thus how an image may be governed by the Euclidean law of proportion. He reassures his readers that the ‘prolix geometric demonstrations’ (59) which he usually gives his friends have been omitted only for the sake of brevity. He recommends a drawing device he refers to as ‘that veil which among my friends I call an intersection’ (68), which we also know as the ‘window’, and in which we would recognise a model of Alberti’s concept of a picture (Fig. 10, above). Furthermore, he describes an easy way of drawing a pavement of square tiles in perspective which, even though it requires no mathematics at all, would be, in his view, sufficient sign of the painter’s learning and the legitimate stage for the painter’s greatest work, namely the istoria.

It is a measure of Alberti’s skill as a propagandist, and of the potential of geometry to short circuit history, that this construction was taken for a mathematically fully rationalised image of space, in Panofsky’s words, ‘an unambiguous and consistent spatial structure of ... infinite extension’. [note 18] It’s hard to say whether Alberti’s gesture towards infinity [note 19] is more sincere than his call for a ‘more sensate wisdom’ (43) in acknowledgement of the painter’s trade. In any case, Alberti is shrewd enough as a painter to avoid absurd labour (infinite regress) and shrewd enough as writer to mask the switches in his text, and, to put it in anachronistic terms, to divert attention from those aspects—the ambiguities and abysses—of the perspective construction, and of projection in general, that would tend to destabilise the epistemological claims of his picture theory. Here, Wittgenstein would suggest a double commentary. In so far as his early ‘picture theory’ of language can be read as paraphrase of Alberti’s theory of pictures, his later investigations provide a searching critique.

Returning at last to Structural Constellations, the ambiguity with which they confront us occurs because we look at them as pictures—after all, we have no third eye to look at them differently, nor any other script for this spectacle (to use Monge’s terms) that could possibly reconcile the apparent contradictions of the trace.

We accept Structural Constellations as pictures despite their puzzling aspects: despite the fact they have no objects. After all, that’s normal for pictures. There is no structural constellation behind the picture as if behind a veil, just as there is no room with an open window and a man and woman posing as artist and model behind Dürer’s recursive depiction of Alberti’s veil (Fig. 10, above), nor any real pavement behind Alberti’s construction (let alone an infinite one). That is to say, there is neither a thing nor a space standing behind the graphic structure that is nonetheless made to stand for (to signify and guarantee) the whole apparatus of projection. The apparatus, that is, which would penetrate and generate the picture, demonstrated by the device I called an automaton (Fig. 3, below), which Dürer said could ‘render anything within reach in correct perspective by means of three threads’. [note 20] Those threads which could be, in Wittgenstein’s image, ‘the feelers of the picture’s elements, with which the picture touches reality’. [note 21]


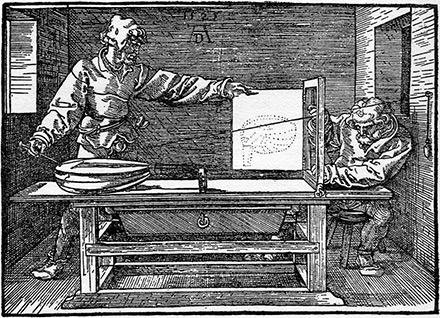
Figure 3 A drawing apparatus from Albrecht Dürer, Underweysung der Messung, 1525. Nuremberg. The thread stretched between the ‘eye’ in the wall and the object on the table intersects a plane described by a frame. The intersection is located by the two moveable threads in the frame and transferred to the hinged picture surface. This ‘automatic’ device excludes the draftsman as viewer, but requires two people to operate it.

Alberti’s construction, under whose sign the picture is to be acknowledged as the trace of appearance—the structure whose intervention, like a map’s grid, suffices to index the surface, and hence to make signs of marks, and indeed, make sense of signs—this structure has no object. Like Alberti’s construction, Structural Constellations inscribe surfaces but reach out to nothing.

However, unlike Alberti’s construction, Structural Constellations exhibit no converging lines, and imply no bundling of rays that would identify or confine the subject whose theoretical eye measures the scene, triangulates image and object, makes them similar, and thus subjects resemblance to geometry. Structural Constellations look, like the technical drawings beloved of the modernists, as if they are organised by a system of parallel projectors rather than ruled from the centre—in Alberti’s language, by ‘the prince of rays’ (48), towards which all others bend.

The notion of parallel projectors unites a range of drawing systems designated as ‘geometrical’ or ‘mechanical’, including architectural plans and elevations [note 22] as well as ‘three-dimensional’ representations, sometimes called ‘projections’, drawn in what is often confusingly called axonometric or isometric ‘perspective’. But such a notion of projectors, it should be emphasised, makes sense only if these drawing conventions are rationalised as projective systems in three dimensions, rather than simply as flat methods, which is how they were normally used. [note 23]

Nineteenth-century pedagogic justifications of such methods did not stop short of origin myths. Pliny’s tale of the girl who traced the outline of her boyfriend’s shadow cast by a lamp, exhibiting central projection, equivalent to perspective—adopted in the eighteenth century as the origin of painting [note 24]—was thus adapted by the architect Karl Friedrich Schinkel. In his version, a draftsman in arcadian sunlight traces the contour of a girl’s shadow, the gender reversal being accompanied by the switch to parallel projectors. [note 25] The everyday teaching of technical drawing tended to explain its methods partly by means of projective geometry—though this was not often of much practical use, it helped support the notion of geometry as higher knowledge and thus secured the institutional hierarchy—and partly by analogy with perspective. The textbooks and manuals propagated a variety of metaphors (‘not without some fantasy or incoherence’, [note 26] as one historian of technical drawing puts it): of an eye removed to infinity, immune from the subjective distortions of perspective, or of a roving eye as it were besieging the object, and from several view points, assembling a representation, no less, of the thing-in-itself, rather than its mere appearance—images which, in the wake of cubism, became the commonplaces of art criticism. [note 27]

The resemblance of Albers’ graphics mobilises the pictorial qualities of technical drawings along with their attendant fantasies, ambiguities and abysses. The numerous working drawings Albers made for Structural Constellations could be the mise en abîme of technical drawing, in so far as they are instructions to manufacturers, such as sign-makers, engravers and steel fabricators, for the production of objects which resemble nothing but technical drawings (Fig. 11, below).


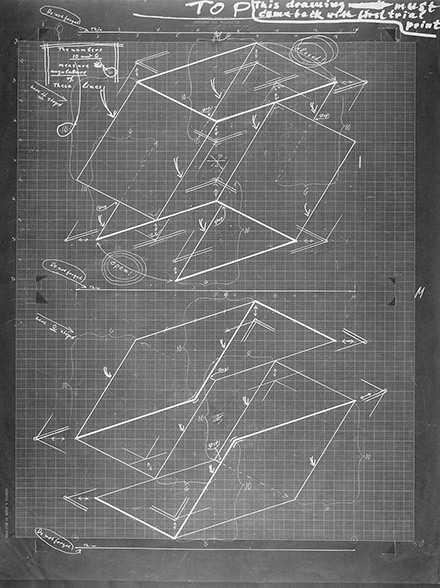
Figure 11: Josef Albers, Two Structural Constellations, c. 1950–60. A copy of a working drawing, 62.5 x 42.7 cm). Bethany, CT: The Josef and Anni Albers Foundation. Albers left many working drawings for engravings, prints and murals, many annotated for himself during the process of refining the designs, or in communication with specialist fabricators. Copyright: The Josef and Anni Albers Foundation (SC: 1239).

It is no accident that the repertoire of illusions once classed as geometric or optical, that came into the possession of experimental psychology in the nineteenth century, is preserved in the language of technical drawing. [note 28] Nor is it an accident that even when they are exhibited as specimens of illusion, such drawings don’t easily shed the connotation of objectivity they inherit from their geometric parentage and which was preserved in the artistic détournement of technical drawing. It should be remembered that the modernists justified the adoption of a technical-geometric idiom exclusively on the grounds it was objective, universal and unequivocal. Later interpretations of modernism highlighting the ambiguity of so-called geometric art owe a debt to Albers, but are usually unwilling to abandon the transcendent promise of geometry. [note 29]

Albers insists—if one may paraphrase Albers’ dictum without the paradox—what you see is what you get, for pictures do not permit a more privileged access to objects than seeing, nor any strict delineation of inspecting a surface from interpreting an image.

To argue, with prolix demonstrations, that Albers’ premise, to draw a straight line, is barely geometrical, is fundamentally, or only primitively so; to argue that his geometry would be more Pythagorean or more Cartesian, is never going to be decisive. The mischief we have to reckon with is that the appearance of geometry is the subterfuge by which Albers absconds from his work. We would search in vain for the location of the authorial eye, the point of view that would authenticate the picture, just as we will not find an object (real or ideal) which could justify the claims or satisfy the desires written into the language of drawing. While the traditional discourse of art history and criticism, notwithstanding its appeals to higher authority, provides no ready-made rationale of interpretation that will not drive us towards paradox and contradiction, Albers has prepared us an autonomous work, because it works without him: an automaton that lies in wait for us.

Albers’ constructions are aptly called constellations. A constellation does not exist in space, but only as the configuration of a surface. A constellation is a sign whose object (in Peirce’s terms, an ‘immediate object’ [note 30]) is contained in and owes its existence to the sign. And does that not imply—does that not interpellate—a subject whose theoretical eye conjures images?

‘L'œil théoricien’ by Hubert Damisch ...
... return... return: Structural Constellations
... return... return: On drawing
... return... return: On theory

Notes

  1. Ludwig Wittgenstein, Tractatus Logico-Philosophicus, trans. by D. F. Pears and B. F. McGuinness, London: Routledge & Kegan Paul, 1961, 57, §5.632 (Logisch-Philosophische Abhandlung, 1921). [back to text]
  2. Hubert Damisch, ‘L’œil théoricien’ in: Josef Albers, Tourcoing: Musée des Beaux Arts, 1988, 11–17. See my translation in this issue of the Journal of Art Historiography, quoted in the following paragraphs. The present article is based on the paper I read (in Damisch’s presence) at the symposium Hubert Damisch: Dialogues with Others, University of Amsterdam, 29 May 2009. I am grateful to Vardan Azatyan and Kent Minturn for their helpful comments on an earlier draft of this essay. [back to text]
  3. See Anthony Auerbach, ‘Structural Constellations: Excursus on the Drawings of Josef Albers c. 1950–1960, with a catalogue of unpublished drawings in the collection of the Josef and Anni Albers Foundation, Connecticut’, PhD Thesis, University of London, 2004). [back to text]
  4. It would not, after all, be justified by the familiar notion of ‘colour space’ which implies only that any set of variables may be expressed as co-ordinates in a space of as many dimensions. [back to text]
  5. ‘The measure of art: The ratio of effort to effect.’ According to Albers’ formulation of the origin, content, measure and aim of art, composed c. 1940. See my translation of Damisch’s ‘L’œil théoricien’ in this issue of the Journal of Art Historiography, note 8; Josef Albers, Search Versus Re-search, Hartford, CT: Trinity College Press, 1969, 10. [back to text]
  6. In 1949, in a review in The Nation, Clement Greenberg compared Albers’ work dismally with the ‘progress’ of younger painters (Adolph Gottlieb and Jackson Pollock), whom Greenberg identified and celebrated as Americans, while characterising ‘Bauhaus modernism’, which he perceived as Albers’s handicap, with epithets stereotypically associated with Germans: ‘Alas, Albers must be accounted another victim of Bauhaus modernism, with its doctrinairism, its static, machine-made, and logical art, its inability to rise above merely decorative motifs.’ 19 February 1949, 222. In 1965, in a private interview, Albers made the comment: ‘This is not the work of angst. [Harold] Rosenberg, via Sartre, thinks everything is angst. I expect the bomb, but without angst.’ Interview with Cecily Sash, transcript in the archives of the Josef and Anni Albers Foundation. [back to text]
  7. The literature on Albers is mainly confined to exhibition catalogues and reviews. The dearth of scholarly interpretation of Albers also suggests that the appeal of Albers’ work is not without its challenges. Damisch is not the only scholar whose interest in Albers was not pursued into an extended study, or whose study was not (yet) published as a book. [back to text]
  8. Pierre Cabanne, Dialogues with Marcel Duchamp, trans. by Ron Padgett, London: Thames & Hudson, 1971, 61. According to the instructions Duchamp sent his sister (1919), the object is supposed to be a geometry textbook. [back to text]
  9. ‘Ce qui défigure la mesure’. Francis Picabia, Poèmes et dessins de la fille née sans mère, Lausanne: Imprimeries Réunies, 1918, 7. [back to text]
  10. Guillaume Apollinaire (1880–1918), French poet, critic and painters’ friend, enthusiast for (modern) geometry as a badge of the avant garde. [back to text]
  11. Galileo Galilei, Discoveries and Opinions of Galileo, trans. by Stillman Drake, Garden City, NY: Doubleday, 1957, 237–238 (Il Saggiatore, 1623). [back to text]
  12. Isaac Newton, The Mathematical Principles of Natural Philosophy, trans. by Andrew Motte, London: Benjamin Motte, 1729, Preface (Philosophiae Naturalis Principia Mathematica, 1687). [back to text]
  13. Immanuel Kant, Critique of Pure Reason, trans. by Norman Kemp Smith, London: MacMillan, 1929, 579 (Kritik der reinen Vernunft, 1781). [back to text]
  14. Sigmund Freud, ‘The Dissection of the Psychical Personality’ in: New Introductory Lectures on Psycho-analysis and Other Works (1932–1936), ed. by James Strachey, London: Hogarth Press, 1964, 72. [back to text]
  15. David Hilbert’s Die Grundlagen der Geometrie (1899) is regarded as the culmination of nineteenth-century progress in axiomatics, which amounted, in Albert Einstein’s words, to ‘the clean separation of logical form from realistic or descriptive contents’. Albert Einstein, Geometrie und Erfahrung, Berlin: Julius Springer, 1921), 4. It is worth emphasising that non-Euclidean geometries stem from an immanent critique of geometry as a formal, deductive system, and not from any ‘scientific’ (empirical) investigation of space. The progress Einstein made in theoretical physics depended on mathematical models conceived independently of reality. That reality agreed with Einstein was received as sensational news when the results of astronomical observations carried out during the solar eclipse of 1919 confirmed how Einstein’s general theory of relativity (1915) predicted the light from distant stars would be ‘bent’ by the sun’s gravity. Whereas, as far as mathematicians were concerned, that didn’t prove anything about geometry (Euclidean or not), the result fired the popular imagination and seemed to confirm what was until then only mathematical rumour and speculation about exotic geometries, propagated as much by literary and theosophist fantasies as by Poincaré’s popular books, not to mention the pretenders to the artistic avant garde. [back to text]
  16. Gaspard Monge, Géométrie descriptive, Paris: Baudouin, 1799, 16. Lacan’s project of geometrising psychoanalysis - the topology he supposed would account for the constitution of the subject - also proceeds from a notion of Descartes’ analytic geometry. Identifying the Freudian with the Cartesian subject, Lacan asks rhetorically, ‘What does that imply? - if not that we are going to be able to start playing with the little letters of algebra, which transform geometry into analysis ... that we can allow ourselves everything as hypothesis of truth.’ Jacques Lacan, Les quatre concepts fondamentaux de la psychanalyse, Paris: Seuil, 1973, 37. [back to text]
  17. Leon Battista Alberti, On Painting, trans. by John R. Spencer, New Haven, CT and London: Yale University Press, 1966, 90 (Della Pittura, 1435). [back to text]
  18. Panofsky does not forget the proviso ‘(within the limits of the “line of sight”)’ - whatever that means. Erwin Panofsky, Perspective as Symbolic Form, trans. by Christopher S. Wood, New York: Zone Books, 1991, 63 (‘Die Perspektive als symbolische Form’, 1927). [back to text]
  19. Quasi persino in infinito: ‘as if [almost even] to infinity’. Alberti, On Painting, 56. [back to text]
  20. Albrecht Dürer, The Painter’s Manual, trans. by Walter L. Strauss, New York: Abaris, 1977, 391. [back to text]
  21. Wittgenstein, Tractatus, 9, §2.1515. [back to text] [back to text]
  22. ‘Orthographic projection’ is often used to refer to to-scale drawings in which ‘views’ of each face of an object are related in a conventional way. The term orthographic survives from Vitruvius’ designation of architectural drawings (ichnographia - plan, orthographia - elevation) although the notion of projection is a later addition. [back to text]
  23. Booker makes the distinction between primary and secondary geometries. The former being the theoretical projective basis for a drawing system understood in three dimensions, the latter being the two-dimensional drawing procedure. Primary geometry for ‘oblique-’, sometimes called ‘axonometric projections’, he says, was a product of the late nineteenth century, ‘invented to account for a secondary geometry which had been in use for ages. True as this projectional system is, it has never yet proved to be of any use. An oblique view of an object can be drawn without any idea of the nature of projection - and even if its form is explained in terms of projection, one will still continue to draw it the same way.’ P. J. Booker, A History of Engineering Drawing, London: Chatto and Windus, 1963, 211–212. [back to text]
  24. The episode in Pliny’s Natural History in fact occurs in connection with the art of modelling portraits (Book 35, chapter 43), but seems to coincide with the beginnings of painting discussed earlier (Book 35, chapter 5). [back to text]
  25. Karl Friedrich Schinkel, Die Erfindung der Malerei, 1830. Gouache, 26 x 29 cm. Wuppertal: Von-der-Heydt-Museum. [back to text]
  26. Yves Deforge, Le Graphisme technique: son histoire et son enseignement, Seyssel: Champ Vallon, 1981, 212. [back to text]
  27. Kahnweiler’s reflections on cubism make explicit reference both to Mongean pedagogy and Kantian philosophy, for instance: ‘no longer bound to the more or less verisimilar optic image which describes the object from a single view point,’ Kahnwieler explains, painting ‘can, in order to give a thorough representation of the object’s primary characteristics, depict them as stereometric drawing on the plane, or through several representations of the same object, can provide an analytical study of that object which the spectator fuses into one again in his mind.’ Furthermore, with a more open treatment, ‘the painter can, if he prefers, also create [...] a synthesis of the object, or in the words of Kant, “put together the various conceptions and comprehend their variety in one perception.”’ Daniel Henry Kahnweiler, The Rise of Cubism, trans. by Henry Aronson, New York: Wittenborn Schultz, 1949, 12 (Der Weg zum Kubismus, 1920, written c. 1915). [back to text]
  28. For instance, the ambiguous ‘Necker cube’, once thought to be an optical phenomenon, came to light in the context of crystallographic diagrams. At the time, crystallography was a new science which depended principally on physical typology and geometric hypothesis, therefore more on drawing than on chemistry as it is understood today. The eponymous Necker was a Professor of Mineralogy. L. A. Necker, ‘Observations on some Remarkable Phænomena seen in Switzerland; and an Optical Phænomenon which Occurs on Viewing a Figure of a Crystal or Geometrical Solid’, The London and Edinburgh Philosophical Magazine and Journal of Science, 3: 1, November 1832, 336. [back to text]
  29. Yve-Alain Bois’ abortive attempts to establish ‘axonometry’ (as it were) as symbolic form would be a case in point. Bois claims undecidability is an inherent property of ‘axonometry’, what he calls the ‘magic of axonometry’s fundamental ambiguity’. Yve-Alain Bois, ‘Metamorphosis of Axonometry’, Daidalos, 1, 15 september 1981, 56 (article later reworked by Bois in two later essays on El Lissitzky, 1988 and 1990). The fact is, all representations are ambiguous if they are deprived of signs sufficient to determine their interpretation, just as all perspectives are anamorphic. [back to text]
  30. ‘We have to distinguish the Immediate Object, which is the Object as the Sign itself represents it, and whose being is thus dependent upon the Representation of it in the Sign, from the Dynamical Object, which is the Reality which by some means contrives to determine the Sign to its Representation.’ Charles S. Peirce, Collected papers of Charles Sanders Peirce, Cambridge: Harvard University Press, 1931, Vol. 4, §536. [back to text]